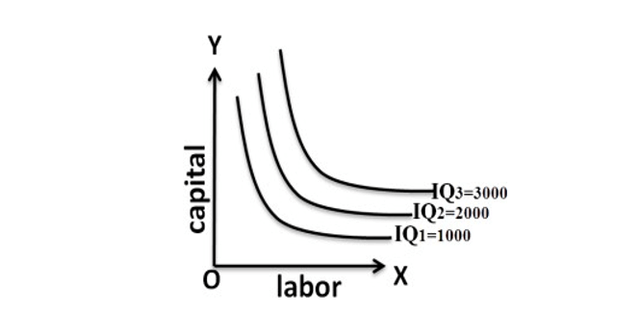
Production Function With Two Variable Inputs
Isoquants analyse and compare the different combinations of capital & labour and output. The term isoquant has its origin from two words “iso” and “quantus”. „iso‟ is a Greek word meaning „equal‟ and „quantus‟ is a Latin word meaning „quantity‟. Isoquant therefore, means equal quantity. An isoquant curve is therefore called as iso-product curve or equal product curve or production indifference curve.
Thus, an isoquant shows all possible combinations of two inputs, which are capable of producing equal or a given level of output. Since each combination yields same output, the producer becomes indifferent towards these combinations.
Assumptions:
- There are only two factors of production, viz. labour and capital.
- The two factors can substitute each other up to certain limit
- The shape of the isoquant depends upon the extent of substitutability of the two inputs.
- The technology is given over a period.
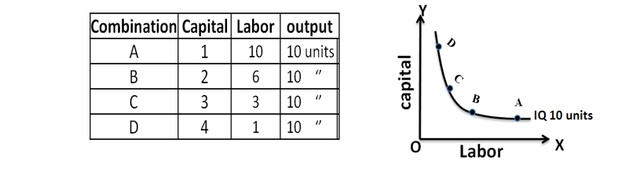
For example:- Now the firm can combine labor and capital in different proportions and can maintain specified level of output say, 10 units of output of a product X. It may combine alternatively as follows: In the below table, combination „A‟ represent 1 unit of capital and 10 units of labour and produces „10‟ units of a product. All other combinations in the table are assumed to yield the same given output of a product say „10‟ units by employing any one of the alternative combinations of the two factors labour and capital. If we plot all these combinations on a paper and join them, we will get a curve called Iso-quant curve as shown below.
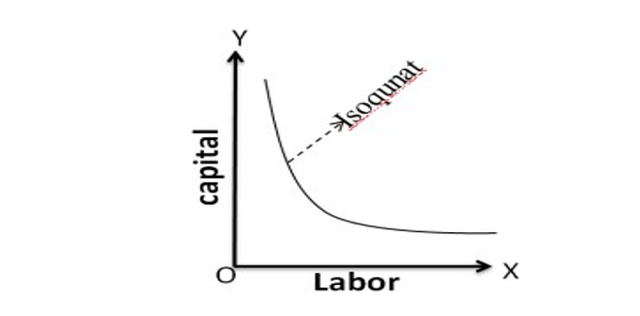
Labour is on the X-axis and capital is on the Y-axis. IQ is the Iso-Quant curve which shows all the alternative combinations A, B, C, D which can produce 10 units of a product.
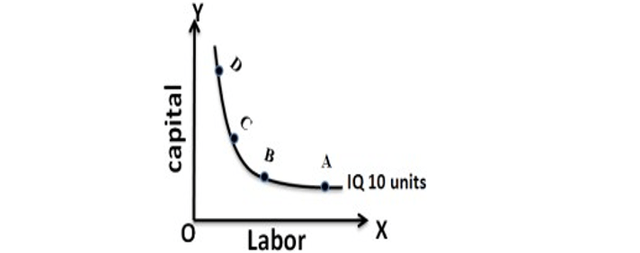
Features of an ISOQUANT:
- Downward sloping: If one of the inputs is reduced, the other input has to be increased. There is no question of increase in both the inputs to yield a given output.
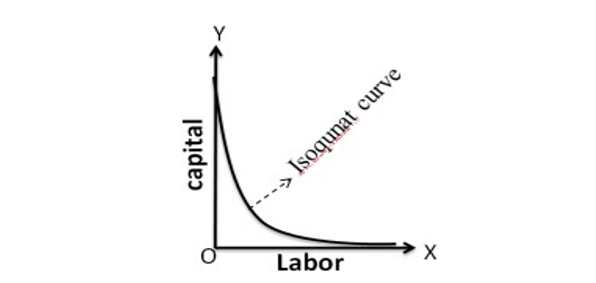
- Don’t touch the axes: The isoquant touches neither X-axis nor Y-axis, as both inputs are required to produce a given product. If an isoquant is touching the X-axis, it means output is possible even by using a factor(Ex: Labor alone without using capital). But, this is unrealistic.
Production Function With Two Variable Inputs
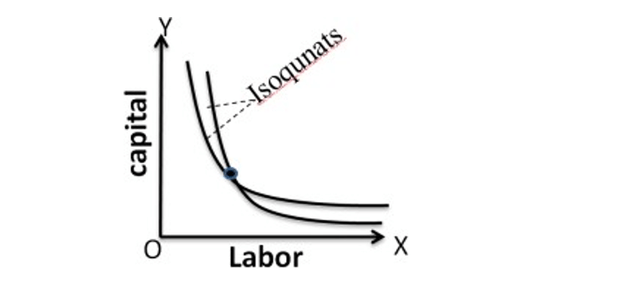
- Don’t intersect: Iso-quants representing different levels of output never intersect or touch or be tangent to each other. If they intersect to each other, they have a common point on them which means that the same amount of labor and capital produce two different levels of output.
Production Function With Two Variable Inputs
- Don’t intersect: Iso-quants representing different levels of output never intersect or touch or be tangent to each other. If they intersect to each other, they have a common point on them which means that the same amount of labor and capital produce two different levels of output.
- Convex to origin: Isoquants are convex to the origin. It is because the inputs factor are not perfect substitutes. One input factor is substituted by other input factor in a decreasing marginal rate. The convexity of isoquant suggests that MRTS is diminishing which means that as quantities of one factor-labor is increased, the less of another factor-capital will be given up, if output level is to be kept constant.
- Upper isoquants represent higher level of output: Each isoquant represents a different quantity of output. Higher isoquants indicate a higher level of output.